Fibonacci Retracements

Traders who use technical analysis are proud of applying scientific methods of observation and borrowing mathematical concepts from science… but then they fall prey to a superstition from the Middle Ages that has never been proven. This is one of the great mysteries of the trading world.

Italian mathematician Leonardo Pisano Bigollo, who lived between 1170 and 1250 in Italy and was nicknamed "Fibonacci" ("Son of Bonacci"), rediscovered a number sequence that had been known in India for centuries before him. The Fibonacci sequence is only one of many, many arithmetic sequences, but it does have some intriguing properties. For one thing, the Fibonacci sequence has a relationship to various phenomena in nature, like the spiral of the nautilus shell, sunflower petals, and so on. Other interesting properties include that it applies equally to negative numbers as to positive ones. Also, every third number is a multiple of 2, every fourth number is a multiple of 3, every fifth number is a multiple of 4, and so on.

The Fibonacci sequence is 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, etc. Each number is the sum of the two preceding numbers. The magic of the Fibonacci sequence is that each number is approximately 1.618 times greater than the preceding number. This ratio forms the basis of the Golden Rectangle used by the ancient Greeks in their buildings and by painters throughout history to size canvases for the most pleasing shape to the human eye. If you divide any number by the one preceding it, you get 61.8. Sometimes, the numbers after the decimal points are not exactly 61.8 − 8 / 13 = 0.6153 and 55 / 89 is 0.6179, for example. The higher you go in the sequence, the closer the number gets to 1.618 or 61.8. Another ratio that is consistent across the entire sequence is the 38.2% ratio, obtained by dividing any number by the number that is three places further on.

In trading, Fibonacci numbers are often used to estimate the amount a move will retrace before resuming. We all know prices do not move in a straight line but rather in a zigzag pattern of two steps forward and one step back. We use Fibonacci ratios to estimate the one step back as a function of the two steps forward. The process entails finding a low and then the next high before a pullback. We assume the pullback or retracement will be in one of the key Fibonacci ratios – 23.6%, 38.2%, or 61.8%. When the price has pulled back as much as 61.8% of the previous up move, we expect it to halt, and that is where support will lie. If not, we expect a dip all the way to the lowest low where the up move began, or a 100% retracement (even though 100 is not a Fibonacci number). Most analysts also include the 50% retracement, an idea that came from an early 20^th-century trader named W.D. Gann, although 50% is not a Fibonacci number, either.

See the chart below, which depicts a picture-perfect retracement of GBP/USD after an up move, first to 50% and then to 38.2%. This chart certainly seems to validate the application of the Fibonacci sequence.

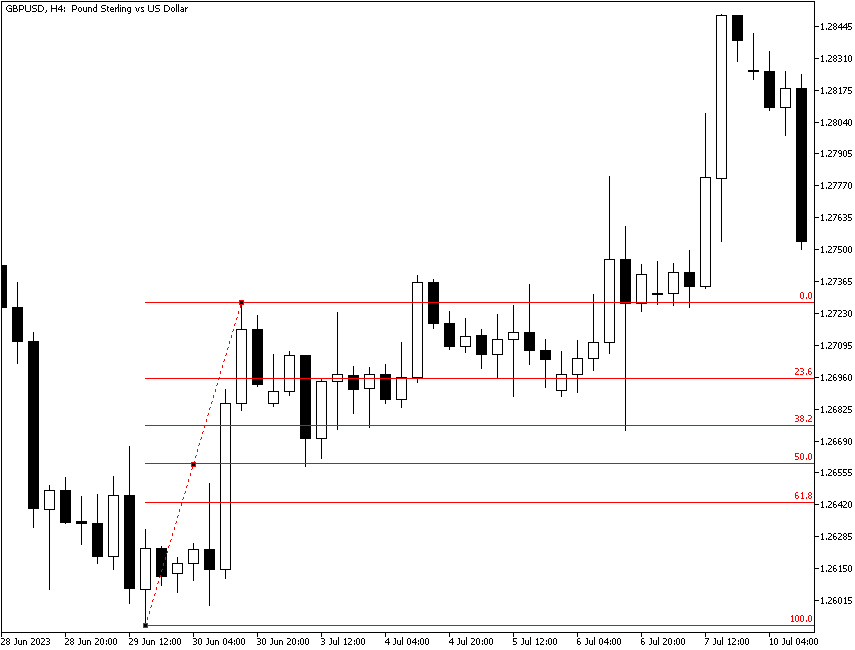
Now compare it to the next chart, where the 61.8% retracement was broken. The price then proceeded to bounce but then go all the way to 100% retracement, only to bounce back once again to meander between the 50% and 61.8% retracement levels, paying no particular heed to them. We may say placing support at the 61.8% retracement level was a failure, but the “theory” worked in the end — the price did proceed to nearly 100% after the 61.8% retracement was broken.

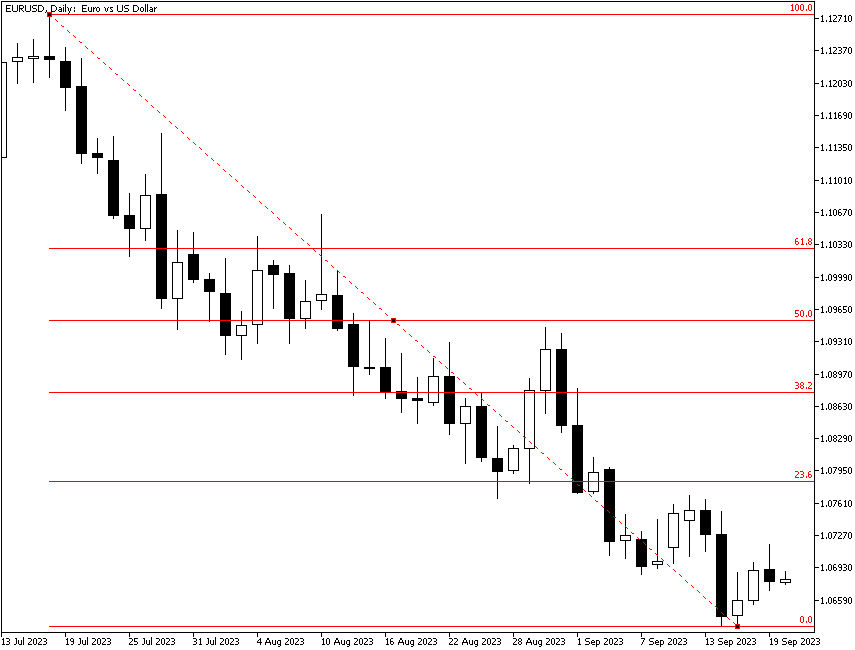
The next figure shows the EUR/USD on the daily timeframe. It is visually obvious that a pretty good place to put a stop in a euro short is just above the 23.6% retracement level.

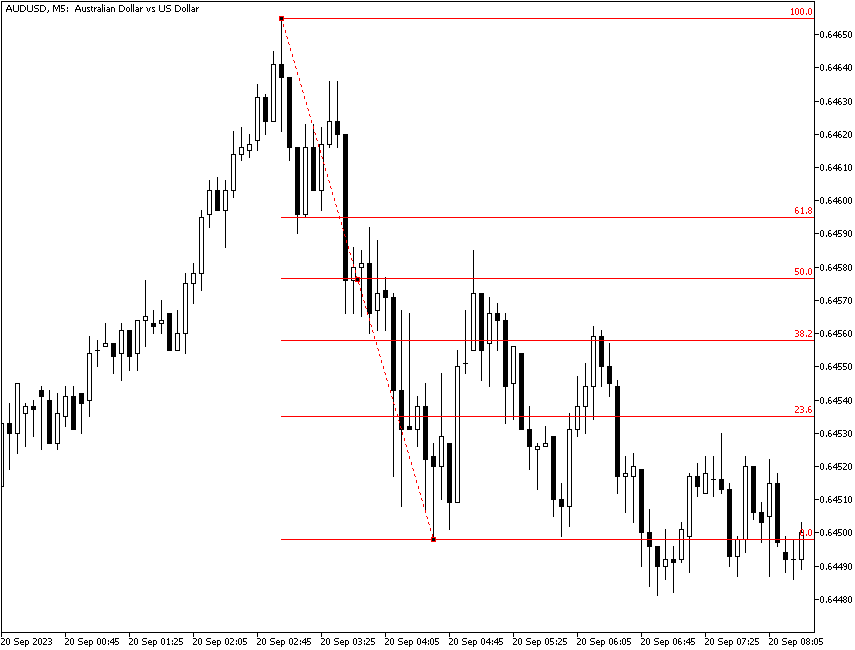
Another example below is showing AUD/USD on a 5-minute basis. The retracement surpasses 50% but does not make it to 61.8%, from which we deduce the rally is failing and we go short. Sure enough, the price goes all the way to the lowest low, our starting point, before starting to move sideways. As a general rule, Fibonacci retracements work somewhat better on shorter timeframes than on daily or weekly ones, which is probably due to so many traders accepting the Fibonacci retracement concept.

There is no reason why securities prices should move in accordance with the Fibonacci sequence any more than they should follow a geometric or triangular sequence or another magical number, pi. All number sequences are “natural law” — and none more so than pi. And yet otherwise rational and scientifically-minded people accept that securities prices, including Forex, do often move according to the Fibonacci sequence. The only sensible explanation is that:

• A sufficient number of instances have been observed in which prices seem to be following the sequence.
• A sufficient number of traders believe or suspect the Fibonacci sequence will work that they apply, and therefore, it does work — a self-fulfilling prophecy.

Faith in the Fibonacci sequence is especially strong in Forex despite no academically respectable studies having ever been done to validate the hypothesis that Forex prices follow the Fibonacci sequence. But because so many Forex traders embrace the concept, it does not pay to get sniffy about the lack of evidence. Many traders draw the Fibonacci lines as a matter of routine, just in case.